

Its three dimensions or the area of its base and its height are known, we are going to look at a question where the volume of the
Volume of a rectangular prism how to#
Now that we have learned how to work out the volume of a rectangular prism when either We find that 𝑉 is greater than 𝑉 , which means that cuboid B is greater in volume than cuboid A. Substituting in the values given in the question, we find that Students must be able to figure the volume of a rectangular prism when given the lengths of the sides, the number of unit cubes in the area of the base. Thus, we know that its volume is 𝑉 = 𝐵 ⋅ ℎ, where 𝐵 is the area of the base and

c mįor cuboid B, we do not have its three dimensions, but we have the area of its base and its height. Substituting in the dimensions given in the question, we find that All its angles are right angles and opposite faces are. Therefore, we can work out its volume with 𝑉 = 𝑙 ⋅ 𝑤 ⋅ ℎ. A rectangular prism is a 3-dimensional object with six rectangular faces. We have the three dimensions of cuboid A We want to compare the volumes of both cuboids. Which cuboid is greater in volume? Answer Cuboid B has a base area of 2 904 cm 2 and a We know it is given by the product of its three dimensions, but we also know that the product of two of its dimensions gives the area of one of its faces.Įxample 4: Finding the Volume of a Rectangular Prism given the Area of Its Base and Its HeightĤ0 cm, and 34 cm. Problem 7: Determine the volume of a rectangular prism if its height is 10 cm and its base length and width are 8 cm and 6 cm, respectively. Hence, the volume of a rectangular prism is 3600 cubic units. Therefore, the man should use the cuboid.īefore we look at other questions, let us observe something interesting about the volume of a rectangular prism. The volume of a rectangular prism l × w × h cubic units. The volume of the cubic box ( 𝑉 ) is smaller than the volume of rice, while the volume of the other box is exactly the volume needed for the rice. The second box is a cube with length 22 cm, We know that the volume of a cuboid is the product of its three dimensions (length, width, and height): 𝑉 = 𝑙 ⋅ 𝑤 ⋅ ℎ = 3 5 ⋅ 2 2 ⋅ 2 1 = 1 6 1 7 0. You can input only integer numbers, decimals or fractions in this online calculator (-2.4, 5/7. The first box is a cuboid of dimensions 35 cm,Ģ2 cm, and 21 cm. We need to compare the volumes of the two boxes in order to decide which one is big enough to contain 16 170 cm 3 of rice. A box has thin walls, so we can consider that its volume is the same as its capacity. The space inside a box is called its capacity, that is, the volume of empty space inside the box that can contain something, here rice. Which box should he use? AnswerĪ box is a cuboid. He has one box which is a cuboid with dimensions of 35 cm,Īnd 21 cm and another box which is a cube with length 22 cm. Download: Volume Activity.Example 3: Comparing the Capacities of BoxesĪ man needs to store 16 170 cm 3 of rice in a container. This activity will provide conversation about estimation, using a 1/8 inch ruler, volume formula, and discussing the difference between estimation and actual measurement. They will then find the actual volume using the volume formula. After finding the estimated volume, students will then use a 1/8th inch ruler to determine the actual length, width, and height of the objects. Students will then determine the volume based on their estimates. Students will estimate length, width, and height of five real-life rectangular prisms.
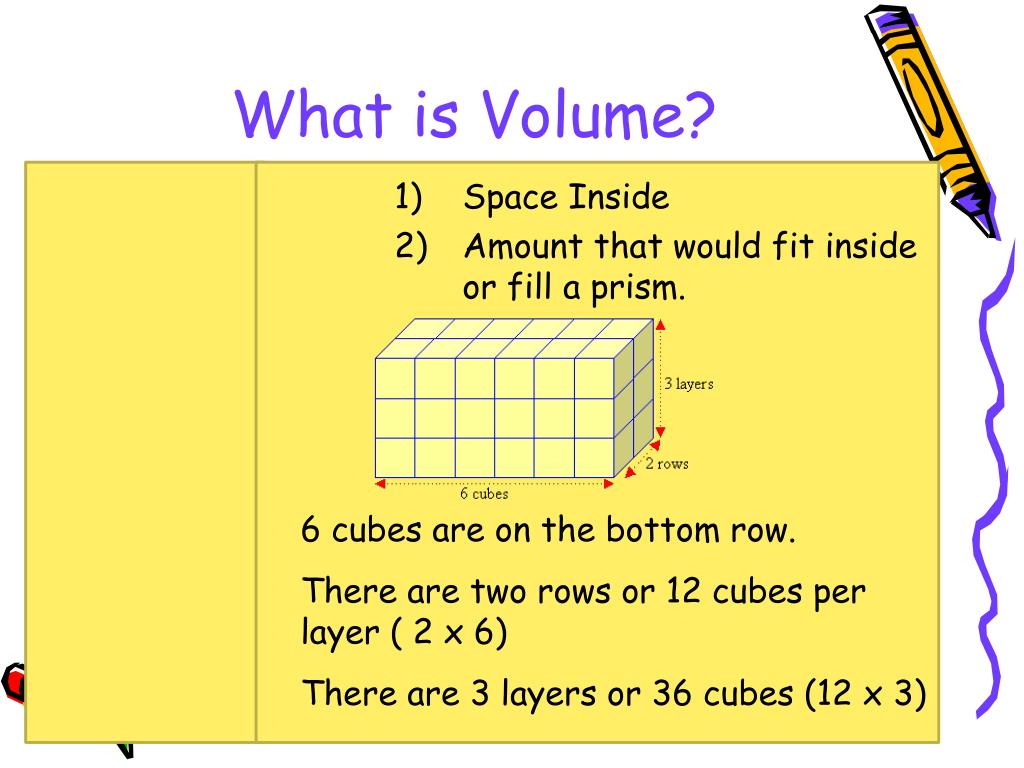
Students will complete a hands-on activity to explore volume. The Rectangular Prism Volume Calculator can instantly calculate the volume of a rectangular prism if you enter in the height, length, and width of the. Typically the various sides of this three dimensional shape are labelled a, b and c. I can find the volume of a right rectangular prism and show that the volume is the same as it would be found by multiplying. Formula for the volume of a rectangular prism The formula to calculate the volume of prism is always the same: Volume prism Area base × Length In this case. Calculating the Volume of a Rectangular Prism. I can relate volume to the operations of multiplication and addition and solve real word involving volume.

I can recognize volumes by counting unit cubes using cubic cm, inches, and feet. UserKristi Hagans Finding the volume of rectangular prisms. Plug in the measures of the length, height and width expressed as decimals in the formula Vlwh and find the volume of each rectangular prism in this set of. Finding the volume of a rectangular prism.


 0 kommentar(er)
0 kommentar(er)
